SOLUTION 23: Compute the area of the region enclosed by the graphs of the equations $ y=x, y=3x, $
and $ x=2 $ . Now see the given graph of the enclosed region.
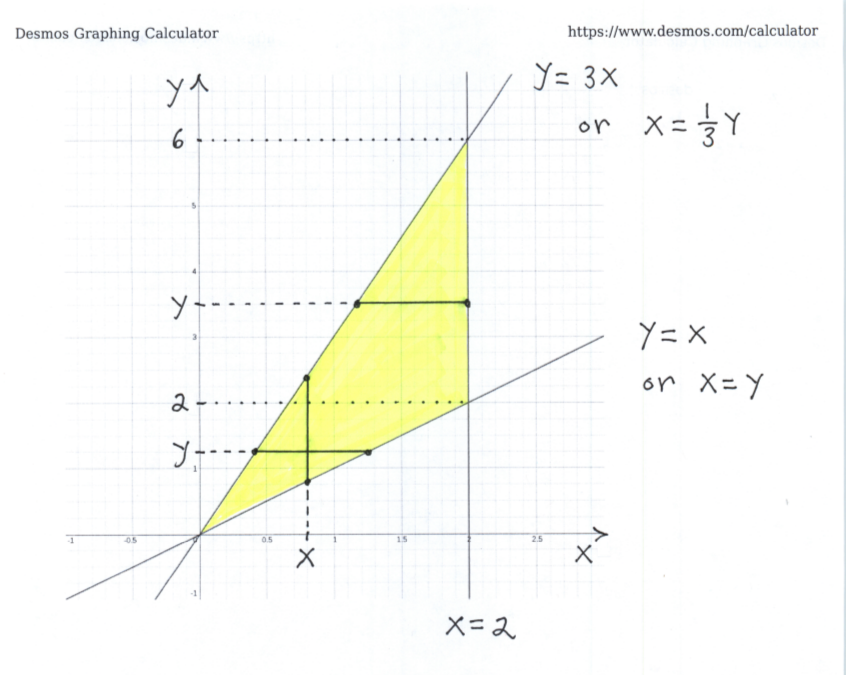
a.) Using vertical cross-sections to describe this region, we get that
$$ 0 \le x \le 2 \ \ and \ \ x \le y \le 3x \ , $$
so that the area of this region is
$$ AREA = \displaystyle{ \int_{0}^{2} (Top \ - \ Bottom) \ dx } $$
$$ = \displaystyle { \int_{0}^{2} ( 3x - x ) \ dx } $$
$$ = \displaystyle { \int_{0}^{2} 2x \ dx } $$
$$ \displaystyle { = (x^2) \Big\vert_{0}^{2} } $$
$$ \displaystyle { = (2)^2 - (0)^2 } $$
$$ = 4 $$
b.) Using horizontal cross-sections to describe this region, which is made up of two smaller regions, we get that
$$ 0 \le y \le 2 \ \ and \ \ \displaystyle{ \frac{1}{3}y } \le x \le y $$
in addition to
$$ 2 \le y \le 6 \ \ and \ \ \displaystyle{ \frac{1}{3}y } \le x \le 2 \ , $$
so that the area of this region is
$$ AREA = \displaystyle{ \int_{0}^{2} ( Right \ - \ Left ) \ dx + \int_{2}^{6} ( Right \ - \ Left ) \ dx } $$
$$ = \displaystyle{ \int_{0}^{2} \Big( y - \frac{1}{3}y \Big) \ dy + \int_{2}^{6} \Big( 2 - \frac{1}{3}y \Big) \ dy } $$
$$ = \displaystyle{ \int_{0}^{2} \Big( \frac{2}{3}y \Big) \ dy + \int_{2}^{6} \Big( 2 - \frac{1}{3}y \Big) \ dy } $$
$$ \displaystyle { = \Big( \frac{y^2}{3} \Big) \Big\vert_{0}^{2}
+ \Big( 2y - \frac{y^2}{6} \Big) \Big\vert_{2}^{6} } $$
$$ \displaystyle { = \Big( \frac{(2)^2}{3} - \frac{(0)^2}{3} \Big)
+ \Big( \Big( 2(6) - \frac{(6)^2}{6} \Big) - \Big( 2(2) - \frac{(2)^2}{6} \Big) \Big) } $$
$$ \displaystyle { = \Big( \frac{4}{3} \Big)
+ \Big( 6 \Big) - \Big( 4 - \frac{2}{3} \Big) } $$
$$ = 4 $$
Click HERE to return to the list of problems.

