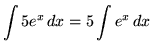
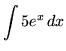Solutions to Integration of Exponential Functions

SOLUTIONS TO INTEGRATION OF EXPONENTIAL FUNCTIONS

SOLUTION 1 : Integrate $\displaystyle{ \int { 5 e^x} \,dx }$ . By formula 1 from the introduction to this section on integrating exponential functions and properties of integrals we get that

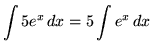
$\displaystyle{ \int { 5 e^x} \,dx } = \displaystyle{ 5 \int { e^x} \,dx }$

$= \displaystyle{ 5 e^x} + C$ .

Click HERE to return to the list of problems.

SOLUTION 2 : Integrate 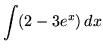$\displaystyle{ \int ( 2-3e^x ) \,dx }$ . By formula 1 from the introduction to this section on integrating exponential functions and properties of integrals we get that

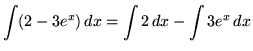
$\displaystyle{ \int ( 2-3e^x ) \,dx } = \displaystyle{ \int 2 \,dx } - \displaystyle{ \int 3e^x \,dx }$

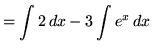
$= \displaystyle{ \int 2 \,dx } - \displaystyle{ 3 \int e^x \,dx }$

$= \displaystyle{ 2x - 3 e^x} + C$ .

Click HERE to return to the list of problems.

SOLUTION 3 : Integrate $\displaystyle{ \int_{0}^{ (1/7) \ln 2 } 14e^{7x} \,dx }$ . Use u-substitution. Let

u = 7x

so that

du = 7 dx ,

(1/7) du = dx .

In addition, the range of x-values is

$x : 0 \longrightarrow (1/7) \ln 2$ ,

so that the range of u-values is

$u : 7(0) \longrightarrow 7(1/7) \ln 2$ ,

$u : 0 \longrightarrow \ln 2$ .

Substitute into the original problem, replacing all forms of x, getting

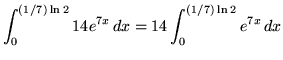
$\displaystyle{ \int_{0}^{ (1/7) \ln 2 } 14e^{7x} \,dx } = \displaystyle{ 14 \int_{0}^{ (1/7) \ln 2 } e^{7x} \, dx }$

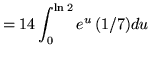
$= \displaystyle{ 14 \int_{0}^{ \ln 2 } e^{u} \, (1/7) du }$

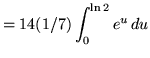
$= \displaystyle{ 14 (1/7)\int_{0}^{ \ln 2 } e^{u} \, du }$

$= \displaystyle{ 2 \int_{0}^{ \ln 2 } e^{u} \, du }$

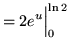
$= \displaystyle{ 2 e^u\Big\vert_{0}^{\ln 2} }$

$= \displaystyle{ 2 e^{\ln 2 } - 2 e^{0} }$

(Recall that $\displaystyle{ e^{ \ln A} = A }$ .)

$= \displaystyle{ 2 (2) - 2(1) }$

= 4 - 2

= 2 .

Click HERE to return to the list of problems.

SOLUTION 4 : Integrate 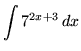$\displaystyle{ \int 7^{2x+3} \,dx }$ . Use u-substitution. Let

u = 2x+3

so that

du = 2 dx ,

(1/2) du = dx .

Substitute into the original problem, replacing all forms of x, getting

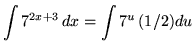
$\displaystyle{ \int 7^{2x+3} \, dx } = \displaystyle{ \int 7^u \, (1/2) du }$

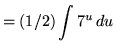
$= \displaystyle{ (1/2) \int 7^u \, du }$

(Now use formula 2 from the introduction to this section on integrating exponential functions.)

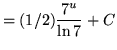
$= (1/2) \displaystyle{ { 7^u \over \ln 7 } + C }$

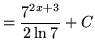
$= \displaystyle{ { 7^{2x+3} \over 2 \ln 7 } + C }$

(Recall that $A \ln B = \ln B^A$ .)

$= \displaystyle{ { 7^{2x+3} \over \ln 7^2 } + C }$

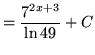$= \displaystyle{ { 7^{2x+3} \over \ln 49 } + C }$ .

Click HERE to return to the list of problems.

SOLUTION 5 : Integrate $\displaystyle{ \int e^{5x} \Big( { e^{2x} \over 7 } + { 3 \over e^{3x} } \Big) \,dx }$ . First, multiply the exponential functions together. The result is

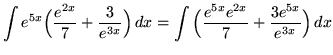
$\displaystyle{ \int e^{5x} \Big( { e^{2x} \over 7 } + { 3 \over e^{3x} } \Big... ...int \Big( { e^{5x} e^{2x} \over 7 } + { 3 e^{5x} \over e^{3x} } \Big) \,dx }$

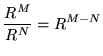(Recall that $\displaystyle{ R^M R^N = R^{M+N}}$ and $\displaystyle{ { R^M \over R^N } = R^{M-N}}$ .)

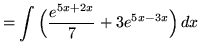
$= \displaystyle{ \int \Big( { e^{5x+2x} \over 7 } + 3 e^{5x-3x} \Big) \,dx }$

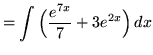
$= \displaystyle{ \int \Big( { e^{7x} \over 7 } + 3 e^{2x} \Big) \,dx }$

(Use the properties of integrals.)

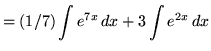
$= \displaystyle{ (1/7) \int e^{7x} \,dx } + \displaystyle{ 3 \int e^{2x} \,dx }$

(Use formula 3 from the introduction to this section on integrating exponential functions.)

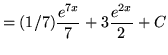
$= \displaystyle{ (1/7) { e^{7x} \over 7 } } + \displaystyle{ 3 { e^{2x} \over 2 } } + C$

$= \displaystyle{ (1/49) e^{7x} } + \displaystyle{ (3/2) e^{2x} } + C$ .

Click HERE to return to the list of problems.

About this document ...

Duane Kouba
1999-05-19