SOLUTION 2: Draw a rectangle with length $x$ and width $y$, and assume each edge of the rectangle is a function of time $t$.
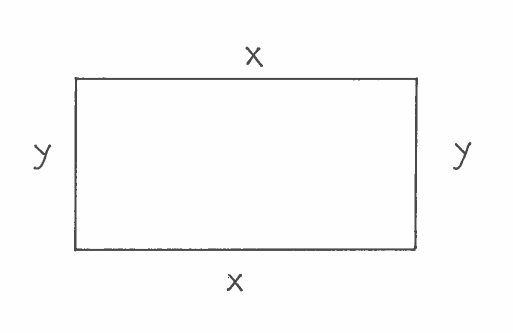
$ \ \ \ \ $ a.) The perimeter of the rectangle is
$$ P=x+x+y+y \ \ \ \ \longrightarrow $$
$$ P = 2x+2y $$
GIVEN: $ \ \ \ \displaystyle{ dx \over dt } = 4 \ ft/min. \ $ and $ \ \displaystyle{ dy \over dt } = -3 \ ft/min. $
FIND: $ \ \ \ \displaystyle{ dP \over dt } $ when $ x=8 \ ft. $ and $ y=5 \ ft. $
Now differentiate the perimeter equation with respect to time $t $ getting
$$ D \{ P \} = D \{2x+2y \} \ \ \ \longrightarrow $$
$$ \displaystyle{ dP \over dt } = 2 \displaystyle{ dx \over dt } + 2 \displaystyle{ dy \over dt } \ \ \ \longrightarrow $$
$\Big($ Now let $\displaystyle{ dx \over dt } = 4 $ and $ \ \displaystyle{ dy \over dt } = -3. \Big) $
$$ \displaystyle{ dP \over dt } = 2 (4) + 2 (-3 ) = 2 \ ft/min. $$
$ \ \ \ \ $ b.) The area of the rectangle is
$$ A= (length)(width) \ \ \ \ \longrightarrow $$
$$ A= xy $$
GIVEN: $ \ \ \ \displaystyle{ dx \over dt } = 4 \ ft/min. $ and $ \displaystyle{ dy \over dt } = - 3 \ ft/min. $
FIND: $ \ \ \ \displaystyle{ dA \over dt } $ when $ x=8 \ ft. $ and $ y=5 \ ft. $
Now differentiate the area equation with repect to time $t $ using the product rule getting
$$ D \{ A \} = D \{xy \} \ \ \ \longrightarrow $$
$$ \displaystyle{ dA \over dt } = x \displaystyle{ dy \over dt } + \displaystyle{ dx \over dt } y \ \ \ \longrightarrow $$
$\Big($ Now let $\displaystyle{ dx \over dt } = 4 $ , $ \ \displaystyle{ dy \over dt } = -3 , x=8, \ $and $y=5. \Big) $
$$ \displaystyle{ dA \over dt } = (8)(-3) + (4)(5) = -4 \ ft^2/min. $$
Click HERE to return to the list of problems.

