SOLUTION 13: Consider the given right triangle with legs $x$ and $y$ and angle $ \theta $ radians, and assume that $ x, y,$ and $ \theta $ are functions of time $t$.
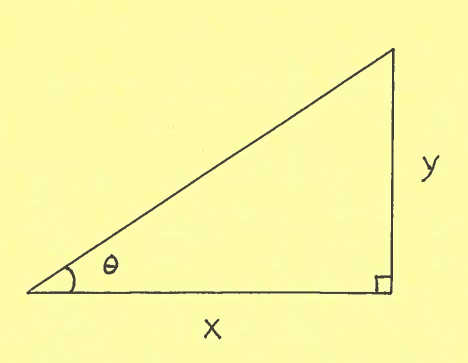
Using trigonometry we can say that
$$ \tan \theta = \displaystyle{ opposite \over adjacent } = { y \over x } \ \ \ \longrightarrow $$
$$ \theta = \arctan \Big({ y \over x } \Big) $$
GIVEN: $ \ \ \ \displaystyle{ dx \over dt } = -3 \ cm/min. $ (It is negative since $x$ is decreasing.) and $ \displaystyle{ dy \over dt } = 4 \ cm/min. $
FIND: $ \ \ \ \displaystyle{ d \theta \over dt } $ when $ \ x =5 \ cm. $ and $ \ y=2 \ cm. $
Recall that
$$ D \{ \arctan f(x) \} = \displaystyle{ 1 \over 1 + (f(x))^2 } \cdot f'(x) $$ Now differentiate the theta equation with respect to time $t $ getting
$$ D \{ \theta \} = D \{ \arctan \Big({ y \over x } \Big) \} \ \ \ \ \longrightarrow $$
$$ \displaystyle{ d \theta \over dt } = \displaystyle{ 1 \over 1 + (y/x)^2 } \cdot D\Big\{ {y \over x} \Big\} \ \ \ \ \longrightarrow $$
$\Big($ Now use the quotient rule.$ \Big)$
$$ \displaystyle{ d \theta \over dt } = \displaystyle{ 1 \over 1 + (y/x)^2 } \cdot { x \displaystyle{ dy \over dt } - y \displaystyle{ dx \over dt } \over x^2 } \ \ \ \ \longrightarrow $$
$$ \displaystyle{ d \theta \over dt } = \displaystyle{ 1 \over 1 + { y^2 \over x^2 } } \cdot { x \displaystyle{ dy \over dt } - y \displaystyle{ dx \over dt } \over x^2 } \ \ \ \ \longrightarrow $$
$$ \displaystyle{ d \theta \over dt } = { x \displaystyle{ dy \over dt } - y \displaystyle{ dx \over dt } \over x^2 +y^2 } \ \ \ \ \longrightarrow $$
$\Big($ Now let $ \displaystyle{ dx \over dt } = -3, \displaystyle{ dy \over dt } = 4, x=5, $ and $ y=2. \Big) $
$$ \displaystyle{ d \theta \over dt } = { (5)(4) - (2) (-3) \over 5^2 + 2^2 } \ \ \ \ \longrightarrow $$
$$ \displaystyle{ d \theta \over dt } = { 26 \over 29 } \ radians/min. $$
Click HERE to return to the list of problems.

