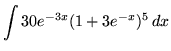Next: About this document ...

SOLUTIONS TO INTEGRATION OF EXPONENTIAL FUNCTIONS



SOLUTION 10 : Integrate $ \displaystyle{ \int 30e^{-3x}(1+3e^{-x})^5 \,dx } $. Use u-substitution. Let

u = 1+3e-x

so that (Don't forget to use the chain rule on e-x.)

du = 3e-x(-1) dx = -3e-x dx ,

or

(-1/3)du = e-x dx .

However, how can we replace the term e-3x in the original problem ? Note that

$ e^{-3x} = e^{(-x)+(-2x)} = e^{-x}e^{-2x} = e^{-x}\big(e^{-x}\big)^2 $ .

From the u-substitution

u = 1+3e-x ,

we can "back substitute" with

e-x = (1/3)(u-1) .

Substitute into the original problem, replacing all forms of x, getting

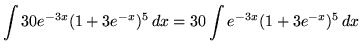
$ \displaystyle{ \int 30e^{-3x}(1+3e^{-x})^5 \,dx } = \displaystyle{ 30 \int e^{-3x}(1+3e^{-x})^5 \,dx } $

$ = \displaystyle{ 30 \int e^{-x}\big(e^{-x}\big)^2 (1+3e^{-x})^5 \,dx } $

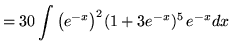
$ = \displaystyle{ 30 \int \big(e^{-x}\big)^2 (1+3e^{-x})^5 \, e^{-x} dx } $

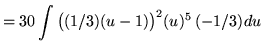
$ = \displaystyle{ 30 \int \big((1/3)(u-1)\big)^2 (u)^5 \, (-1/3) du } $

(Recall that (AB)C = AC BC .)

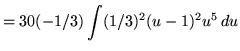
$ = \displaystyle{ 30(-1/3) \int (1/3)^2(u-1)^2 u^5 \, du } $

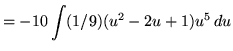
$ = \displaystyle{ -10 \int (1/9)(u^2-2u+1)u^5 \, du } $

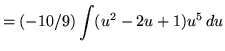
$ = \displaystyle{ (-10/9) \int (u^2-2u+1)u^5 \, du } $

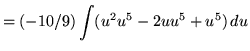
$ = \displaystyle{ (-10/9) \int (u^2u^5-2uu^5+u^5) \, du } $

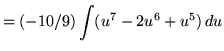
$ = \displaystyle{ (-10/9) \int (u^7-2u^6+u^5) \, du } $

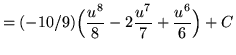
$ = \displaystyle{ (-10/9) \Big( {u^8 \over 8 } -2 { u^7 \over 7 } + {u^6 \over 6} \Big) } + C $

$ = \displaystyle{ (-10/72) u^8 - (-20/63) u^7 + (-10/54) u^6 } + C $

$ = \displaystyle{ (-5/36) (1+3e^{-x})^8 + (20/63) (1+3e^{-x})^7 + (-5/27) (1+3e^{-x})^6 } + C $ .

Click HERE to return to the list of problems.




SOLUTION 11 : Integrate $ \displaystyle{ \int { 8e^x(3+e^x) \over \sqrt{ e^{2x}+6e^x+ 1 } } \,dx } $ . Use u-substitution. Let

u = e2x+6ex+ 1

so that (Don't forget to use the chain rule on e2x.)

du = (2e2x+6ex) dx

= (2ex+x+6ex) dx

= (2exex+6ex) dx

= 2ex(ex+3) dx

= 2ex(3+ex) dx

or

(1/2) du = ex(3+ex) dx .

Substitute into the original problem, replacing all forms of x, getting

$ \displaystyle{ \int { 8e^x(3+e^x) \over \sqrt{ e^{2x}+6e^x+ 1 } } \,dx } = \displaystyle{ 8 \int { 1 \over \sqrt{ e^{2x}+6e^x+ 1 } } \, e^x(3+e^x)dx }$

$ = \displaystyle{ 8 \int { 1 \over \sqrt{u} } \, (1/2) du } $

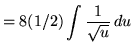
$ = \displaystyle{ 8(1/2) \int { 1 \over \sqrt{u} } \, du } $

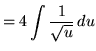
$ = \displaystyle{ 4 \int { 1 \over \sqrt{u} } \, du } $

(Do not make the following VERY COMMON MISTAKE : 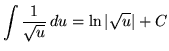$ \displaystyle{ \int { 1 \over \sqrt{u} } \, du } = \displaystyle{ \ln \vert \sqrt{u}\vert } + C $ . Why is this INCORRECT ?)

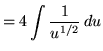
$ = \displaystyle{ 4 \int { 1 \over u^{1/2} } \, du } $

$ = \displaystyle{ 4 \int u^{-1/2} \, du } $

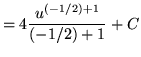
$ = \displaystyle{ 4 { u^{(-1/2) + 1} \over {(-1/2) + 1} } } + C $

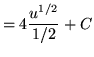
$ = \displaystyle{ 4 { u^{1/2} \over {1/2} } } + C $

$ = \displaystyle{ 4(2) u^{1/2} } + C $

$ = \displaystyle{ 8 (e^{2x}+6e^x+ 1)^{1/2} } + C $

$ = \displaystyle{ 8 \sqrt{e^{2x}+6e^x+ 1 } } + C $ .

Click HERE to return to the list of problems.




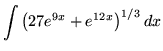SOLUTION 12 : Integrate $ \displaystyle{ \int \big( 27e^{9x}+e^{12x} \big)^{1/3} \,dx } $. First, factor out e9x from inside the parantheses. Then

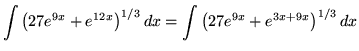
$ \displaystyle{ \int \big( 27e^{9x}+e^{12x} \big)^{1/3} \,dx } = \displaystyle{ \int \big( 27e^{9x}+e^{3x+9x} \big)^{1/3} \,dx } $

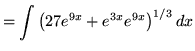
$ = \displaystyle{ \int \big( 27e^{9x}+e^{3x}e^{9x} \big)^{1/3} \,dx } $

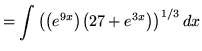
$ = \displaystyle{ \int \big( \big(e^{9x}\big)\big( 27+e^{3x} \big) \big)^{1/3} \,dx } $

(Recall that (AB)C = AC BC .)

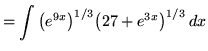
$ = \displaystyle{ \int \big(e^{9x}\big)^{1/3}\big( 27+e^{3x} \big)^{1/3} \,dx } $

(Recall that (AB)C = ABC .)

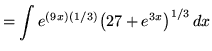
$ = \displaystyle{ \int e^{(9x)(1/3)} \big( 27+e^{3x} \big)^{1/3} \,dx } $

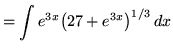$ = \displaystyle{ \int e^{3x} \big( 27+e^{3x} \big)^{1/3} \,dx } $ .

Now use u-substitution. Let

u = 27+e3x

so that (Don't forget to use the chain rule on e3x.)

du = 3e3x dx ,

or

(1/3) du = e3x dx .

Substitute into the original problem, replacing all forms of x , and getting

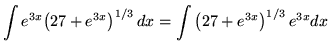
$ \displaystyle{ \int e^{3x} \big( 27+e^{3x} \big)^{1/3} \,dx } = \displaystyle{ \int \big( 27+e^{3x} \big)^{1/3} \, e^{3x}dx } $

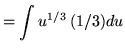
$ = \displaystyle{ \int u^{1/3} \, (1/3) du } $

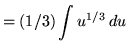
$ = \displaystyle{ (1/3) \int u^{1/3} \, du } $

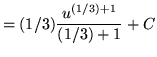
$ = \displaystyle{ (1/3) { u^{(1/3)+1} \over (1/3)+1 } } + C $

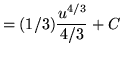
$ = \displaystyle{ (1/3) { u^{4/3} \over 4/3} } + C $

$ = \displaystyle{ (1/3)(3/4)(27+e^{3x})^{4/3} } + C $

$ = \displaystyle{ (1/4)(27+e^{3x})^{4/3} } + C $ .

Click HERE to return to the list of problems.




Duane Kouba
1999-05-19