$polyhedron$

$LattE$

background

Minkowski's Theorem

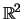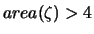Theorem 1 Let $\zeta$ be a convex set in ${\mathbb{R}}^2$ , with $\zeta$ centrally symmetric and $area(\zeta)>4$ . Then $\zeta$ must contain a lattice point besides

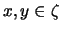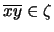Definition 2 A set is convex whenever $x,y\in\zeta$ implies $\overline{xy}\in\zeta$ .

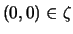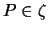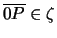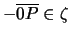Definition 3 An object $\zeta$ is centrally symmetric if whenever $(0,0)\in\zeta$ and $P\in\zeta$ implies $\overline{0P}\in\zeta$ and $-\overline{0P}\in\zeta$ .

1. Take $\frac{1}{2}\zeta=\zeta'$

$\includegraphics[width=2 in]{blob.eps}$

2. Assign a copy of $\zeta'$ to each lattice point in an $n\times n$ grid.

$\includegraphics[width=3 in]{nxn.eps}$

3. From the above figure we can see $area(\zeta')>1$ , thus the area covered by the set s is .

4. In fact, all objects cover more (go over a bit). All objects are contained in a square of side , where is the maximum distance from the center to any point of $\zeta'$ .

$\includegraphics[width=2.75 in]{n2s.eps}$

5. Now we want to show the inequality

$\displaystyle (n+1)^2area(\zeta')>(n+2s)^2$

to be true. CLAIM:

$\displaystyle (n+1)^2(area(\zeta'))-(n+2s)^2$	$\displaystyle =$	$\displaystyle (area(\zeta')-1)(n^2+2n)(area(\zeta)-2s)$
		$\displaystyle {} +area(\zeta')-4s^2$
	$\displaystyle >$	$\displaystyle 0.$

Since $area(\zeta)>1$ implies the leading coefficient is positive for large

, if we choose

large then we're done! Conclusion, the blobs will overlap for some pair of blobs, but by translating $\zeta'$ and another blob $\zeta''$ cross too! (All is being repeated).

6. Let be in the intersection of $\zeta', \zeta''$ . Then

$\displaystyle (a'',b'')=(a',b')+(p,q),$ where $\displaystyle (a',b')\in\zeta'.$

Note that $(-a',-b')\in\zeta'$ as well.

$\includegraphics[width=2 in]{overlap.eps}$

Consider the midpoint of

and

, then $(\frac{p}{2},\frac{q}{2})\in\zeta'\Rightarrow(p,q)\in\zeta$ .

QED

• Mathematics • The University of California, Davis •
• One Shields Avenue • Davis, CA 95616 •