Shell Method Solution 7

Processing math: 100%

Solution a.): Here is a carefully labeled sketch of the region with a shell marked on the

$x$ -axis at

$x$ . The shell has radius

$r$ , measured from the

$y$ -axis, and height

$h$ , taken parallel to the

$y$ -axis at

$x$ . It is IMPORTANT to mark ALL of

$x$ ,

$r$ , and

$h$ in the sketch of the region !!!

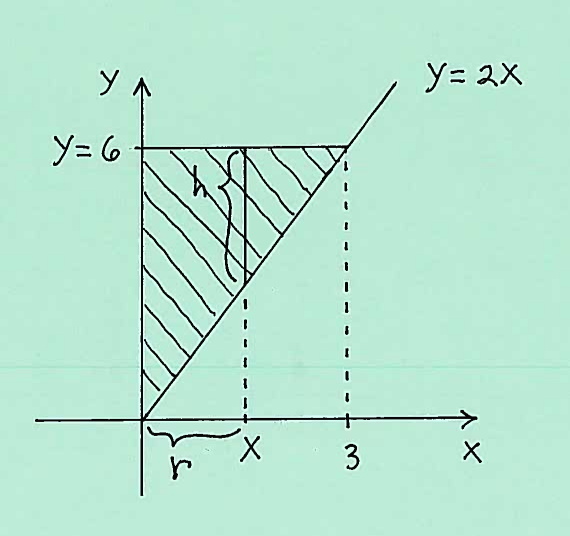
tex2html_wrap_inline125

Thus the total volume of this Solid of Revolution is

$Volume = 2 \pi \int_{0}^{3} (radius)(height) \ dx = 2 \pi \int_{0}^{3} rh \ dx$

$= 2 \pi \int_{0}^{3} (x)(6-2x) \ dx$

Solution b.): IMPORTANT CHANGE: Because we are revolving the region about the

$x$ -axis, we must mark a shell on the

$y$ -axis at

$y$ !!! The shell has radius

$r$ , measured from the

$x$ -axis, and height

$h$ , taken parallel to the

$x$ -axis at

$y$ . It is IMPORTANT to mark ALL of

$y$ ,

$r$ , and

$h$ in the sketch of the region !!!

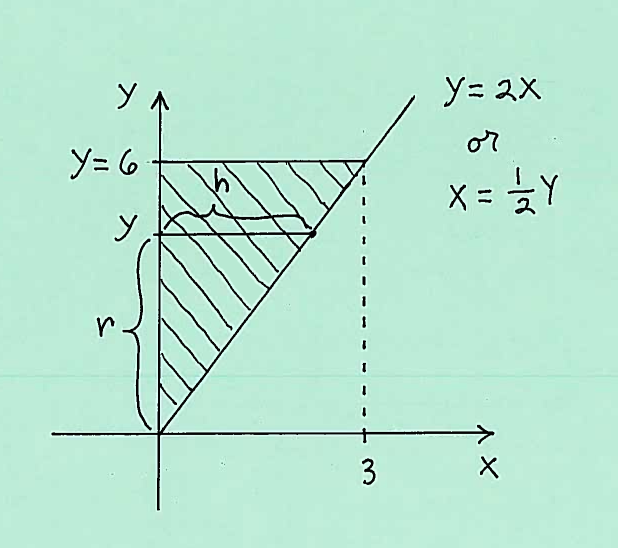
tex2html_wrap_inline125

Thus the total volume of this Solid of Revolution is

$Volume = 2 \pi \int_{0}^{6} (radius)(height) \ dy = 2 \pi \int_{0}^{6} rh \ dy$

$= 2 \pi \int_{0}^{6} (y)({1 \over 2}y) \ dy$

Solution c.): Here is a carefully labeled sketch of the region with a shell marked on the

$x$ -axis at

$x$ . The shell has radius

$r$ , measured from the line

$x=-1$ , and height

$h$ , taken parallel to the

$y$ -axis at

$x$ . It is IMPORTANT to mark ALL of

$x$ ,

$r$ , and

$h$ in the sketch of the region !!!

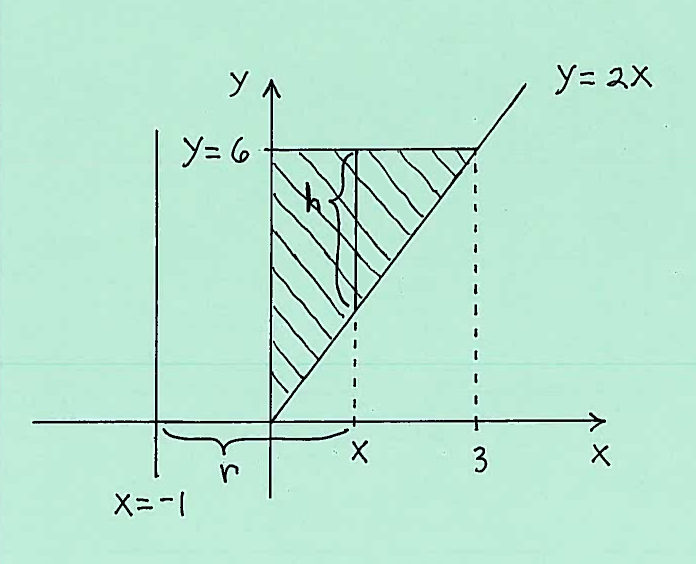
tex2html_wrap_inline125

Thus the total volume of this Solid of Revolution is

$Volume = 2 \pi \int_{0}^{3} (radius)(height) \ dx = 2 \pi \int_{0}^{3} rh \ dx$

$= 2 \pi \int_{0}^{3} (x-(-1))(6-2x) \ dx$

$= 2 \pi \int_{0}^{3} (x+1)(6-2x) \ dx$

Solution d.): Here is a carefully labeled sketch of the region with a shell marked on the

$x$ -axis at

$x$ . The shell has radius

$r$ , measured from the line

$x=5$ , and height

$h$ , taken parallel to the

$y$ -axis at

$x$ . It is IMPORTANT to mark ALL of

$x$ ,

$r$ , and

$h$ in the sketch of the region !!!

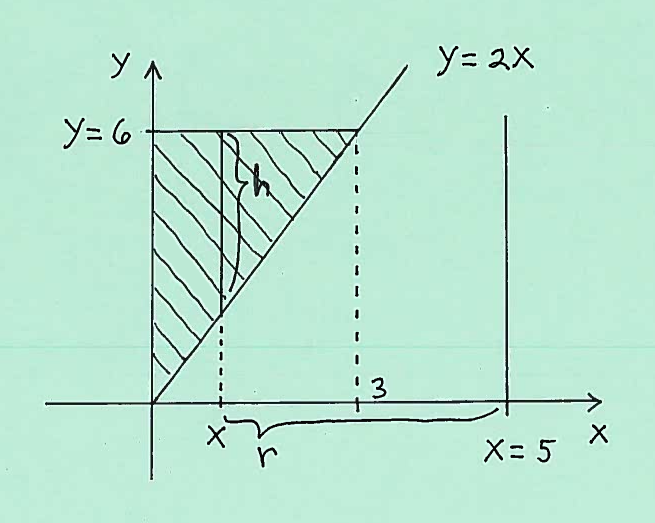
tex2html_wrap_inline125

Thus the total volume of this Solid of Revolution is

$Volume = 2 \pi \int_{0}^{3} (radius)(height) \ dx = 2 \pi \int_{0}^{3} rh \ dx$

$= 2 \pi \int_{0}^{3} (5-x)(6-2x) \ dx$

Solution e.): IMPORTANT CHANGE: Because we are revolving the region about the

$x$ -axis, we must mark a shell on the

$y$ -axis at

$y$ !!! The shell has radius

$r$ , measured from the line

$y=7$ , and height

$h$ , taken parallel to the

$x$ -axis at

$y$ . It is IMPORTANT to mark ALL of

$y$ ,

$r$ , and

$h$ in the sketch of the region !!!

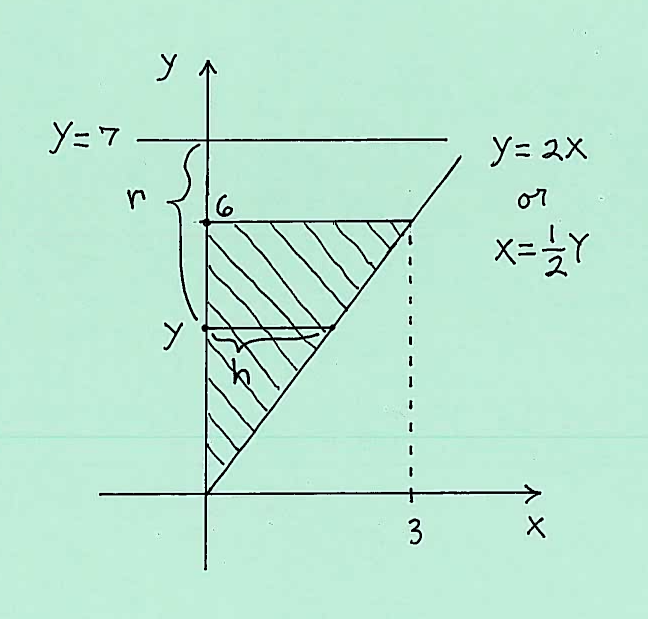
tex2html_wrap_inline125

Thus the total volume of this Solid of Revolution is

$Volume = 2 \pi \int_{0}^{6} (radius)(height) \ dy = 2 \pi \int_{0}^{6} rh \ dy$

$= 2 \pi \int_{0}^{6} (7-y)( {1 \over 2} y) \ dy$

Solution f.): IMPORTANT CHANGE: Because we are revolving the region about the

$x$ -axis, we must mark a shell on the

$y$ -axis at

$y$ !!! The shell has radius

$r$ , measured from the line

$y=-2$ , and height

$h$ , taken parallel to the

$x$ -axis at

$y$ . It is IMPORTANT to mark ALL of

$y$ ,

$r$ , and

$h$ in the sketch of the region !!!

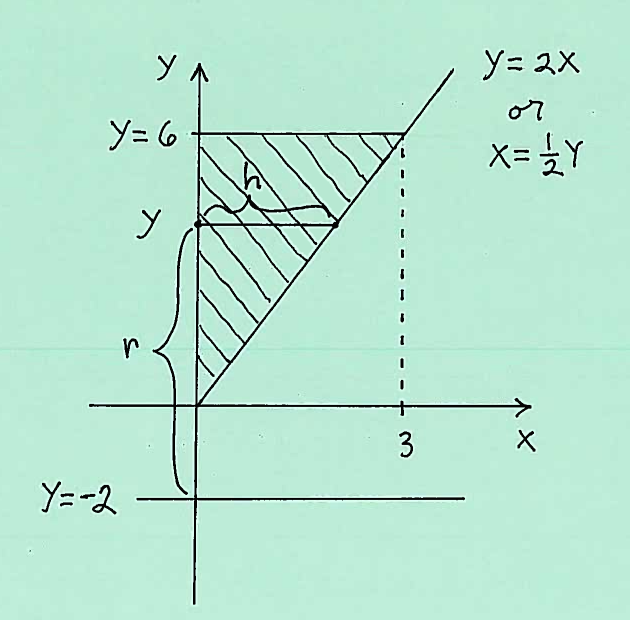
tex2html_wrap_inline125

Thus the total volume of this Solid of Revolution is

$Volume = 2 \pi \int_{0}^{6} (radius)(height) \ dy = 2 \pi \int_{0}^{6} rh \ dy$

$= 2 \pi \int_{0}^{6} (y-(-2))({1 \over 2}y) \ dy$

$= 2 \pi \int_{0}^{6} (y+2)({1 \over 2}y) \ dy$

Click HERE to return to the list of problems.