Next: About this document ...

SOLUTIONS TO TRIGONOMETRIC INTEGRALS



SOLUTION 1 : Integrate $ \displaystyle{ \int { \sin {3x} } \,dx } $ . Use u-substitution. Let

$ u = 3x $

so that

$ du = 3 dx $ ,

or

$ (1/3) du = dx $ .

Substitute into the original problem, replacing all forms of $ x $, getting

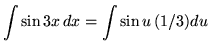
$ \displaystyle{ \int { \sin {3x} } \,dx } = \displaystyle{ \int { \sin u } \,(1/3)du } $

$ = \displaystyle{ (1/3) \int { \sin u } \,du } $

(Use antiderivative rule 2 from the beginning of this section.)

$ = (1/3)\displaystyle{ (-\cos{u}) + C } $

$ = -\displaystyle{ {1 \over 3}\cos{3x} + C } $ .

Click HERE to return to the list of problems.




SOLUTION 2 : Integrate 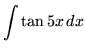$ \displaystyle{ \int { \tan{5x} } \,dx } $ . Use u-substitution. Let

$ u = 5x $

so that

$ du = 5 dx $ ,

or

$ (1/5) du = dx $ .

Substitute into the original problem, replacing all forms of $ x $, getting

$ \displaystyle{ \int {\tan{5x} } \,dx } = \displaystyle{ \int {\tan{u}} \,(1/5)du } $

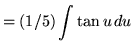
$ = \displaystyle{ (1/5) \int {\tan{u}} \, du } $

(Use antiderivative rule 7 from the beginning of this section.)

$ = \displaystyle{{1 \over 5} \ln{\vert \sec u \vert} + C } $

$ = \displaystyle{{1 \over 5} \ln{\vert \sec {5x} \vert} + C } $ .

Click HERE to return to the list of problems.




SOLUTION 3 : Integrate 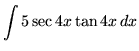$ \displaystyle{ \int{ 5 \sec{4x} \tan{4x} } \,dx } $ . Use u-substitution. Let

$ u = 4x $

so that

$ du = 4 dx $ ,

or

$ (1/4) du = dx $ .

Substitute into the original problem, replacing all forms of $ x $, getting

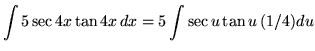
$ \displaystyle{ \int { 5 \sec{4x} \tan{4x} } \,dx } = \displaystyle{ 5\int {\sec{u} \tan{u}} \,(1/4) du } $

$ = \displaystyle{ {5 \over 4 }\int {\sec{u} \tan{u}} \,du } $

(Use antiderivative rule 5 from the beginning of this section.)

$ = \displaystyle{{5 \over 4} \sec u + C } $

$ = \displaystyle{ { {5 \over 4}\sec {4x}} + C } $ .

Click HERE to return to the list of problems.




SOLUTION 4 : Integrate 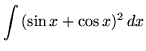$ \displaystyle{ \int { (\sin x + \cos x)^2 } \,dx } $ . Begin by squaring the function, getting

$ \displaystyle{ \int { (\sin x + \cos x)^2 } \,dx } = \displaystyle{ \int { (\sin^2 x + 2 \sin x \cos x + \cos^2 x)} \,dx }$

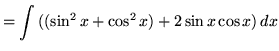
$ = \displaystyle{ \int { ( (\sin^2 x + \cos^2 x) + 2 \sin x \cos x )} \,dx }$

(Use trig identity A from the beginning of this section.)

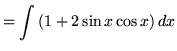
$ = \displaystyle{ \int { (1 + 2 \sin x \cos x )} \,dx } $

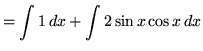
$ = \displaystyle{ \int { 1 } \,dx + \int { 2 \sin x \cos x } \,dx } $

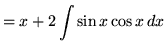$ = \displaystyle{ x + 2 \int { \sin x \cos x } \,dx }$ .

Now use u-substitution. Let

$ u = \sin x $

so that

$ du = \cos x \ dx $ .

Substitute into the original problem, replacing all forms of x, getting

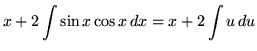
$ \displaystyle{ x + 2 \int { \sin x \cos x } \,dx } = \displaystyle{ x + 2\int { u } \, du } $

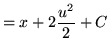
$ = \displaystyle{ x + 2 { u^2 \over 2} + C } $

$ = \displaystyle{ x + u^2 + C } $

$ = \displaystyle{ x + \sin^2 x + C } $ .

Click HERE to return to the list of problems.




SOLUTION 5 : Integrate 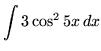$ \displaystyle{ \int { 3 \cos^2 5x } \,dx } $ . First use trig identity C from the beginning of this section, getting

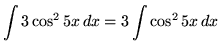
$ \displaystyle{ \int { 3 \cos^2 5x } \,dx } = \displaystyle{ 3 \int { \cos^2 5x } \,dx }$

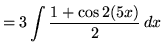
$ = \displaystyle{ 3 \int { 1 + \cos 2(5x) \over 2} \,dx } $

$ = \displaystyle{ (3/2) \int ( 1 + \cos 10x ) \,dx } $

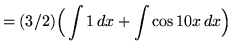
$ = \displaystyle{ (3/2) \Big( \int 1 \,dx + \int \cos 10x \,dx \Big) } $

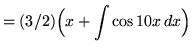$ = \displaystyle{ (3/2) \Big(x + \int \cos 10x \,dx \Big) } $ .

Now use u-substitution. Let

$ u = 10x $

so that

$ du = 10 dx $ ,

or

$ (1/10) du = dx $ .

Substitute into the original problem, getting

$ \displaystyle{ (3/2) \Big(x + \int \cos 10x \,dx \Big) } = \displaystyle{ (3/2) \Big(x + \int \cos u \, (1/10) du \Big) } $

$ = \displaystyle{ (3/2) \Big(x + (1/10) \int \cos u \, du \Big) } $

(Use antiderivative rule 1 from the beginning of this section.)

$ = \displaystyle{ (3/2) \Big(x + (1/10) ( \sin u + C ) \Big) } $

$ = \displaystyle{ (3/2) x + (3/20) ( \sin u + C ) } $

(Combine constant $ 3/20 $ with $ C $ since $ C $ is an arbitrary constant.)

$ = \displaystyle{ (3/2) x + (3/20) \sin u + C } $

$ = \displaystyle{ (3/2) x + (3/20) \sin 10x + C } $ .

Click HERE to return to the list of problems.




SOLUTION 6 : Integrate 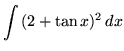$ \displaystyle{ \int { (2 + \tan x)^2 } \,dx } $ . Begin by squaring the function, getting

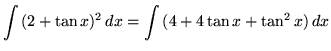
$ \displaystyle{ \int { (2 + \tan x)^2 } \,dx } = \displaystyle{ \int { (4 +4\tan x + \tan^2 x ) } \,dx } $

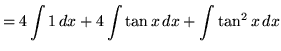
$ = \displaystyle{ 4 \int 1 \,dx + 4 \int { \tan x } \,dx + \int { \tan^2 x } \,dx } $

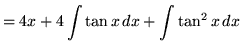
$ = \displaystyle{ 4x + 4 \int { \tan x } \,dx + \int { \tan^2 x } \,dx } $

(Use antiderivative rule 7 from the beginning of this section on the first integral and use trig identity F from the beginning of this section on the second integral.)

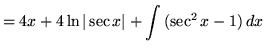
$ = \displaystyle{ 4x + 4 \ln\vert\sec x\vert + \int { ( \sec^2 x - 1 ) } \,dx } $

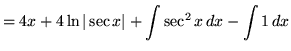
$ = \displaystyle{ 4x + 4 \ln\vert\sec x\vert + \int { \sec^2 x } \,dx - \int { 1 } \,dx } $

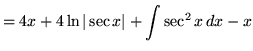
$ = \displaystyle{ 4x + 4 \ln\vert\sec x\vert + \int { \sec^2 x } \,dx - x } $

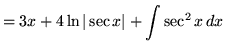
$ = \displaystyle{ 3x + 4 \ln\vert\sec x\vert + \int { \sec^2 x } \,dx } $

(Now use antiderivative rule 3 from the beginning of this section.)

$ = \displaystyle{ 3x + 4 \ln{\vert\sec x\vert} + \tan x + C} $ .

Click HERE to return to the list of problems.




SOLUTION 7 : Integrate 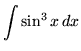$ \displaystyle{ \int \sin^3 x \,dx } $ . First rewrite the function (Recall that $ A^m A^n = A^{m+n} $ .), getting

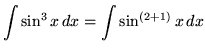
$ \displaystyle{ \int \sin^3 x \, dx } = \displaystyle{ \int \sin^{(2+1)} x \,dx } $

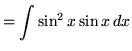
$ = \displaystyle{ \int \sin^2 x \sin x \, dx } $

(Now use trig identity A from the beginning of this section.)

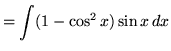
$ = \displaystyle{ \int (1 - \cos^2 x) \sin x \, dx } $

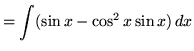
$ = \displaystyle{ \int ( \sin x - \cos^2 x \sin x ) \, dx } $

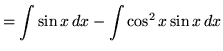
$ = \displaystyle{ \int \sin x \, dx - \int \cos^2 x \sin x \, dx } $

(Use antiderivative rule 2 from the beginning of this section on the first integral.)

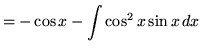$ = - \cos x - \displaystyle{ \int \cos^2 x \sin x \, dx } $ .

Now use u-substitution. Let

$ u = \cos x $

so that

$ du = - \sin x \ dx $ ,

or

$ (-1) du = \sin x \ dx $ .

Substitute into the original problem, replacing all forms of $ x $, getting

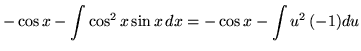
$ - \cos x - \displaystyle{ \int \cos^2 x \sin x \, dx } = - \cos x - \displaystyle{ \int u^2 \, (-1) du } $

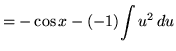
$ = - \cos x - (-1) \displaystyle{ \int u^2 \, du } $

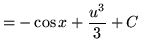
$ = - \cos x + \displaystyle{ u^3 \over 3 } + C $

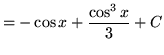$ = - \cos x + \displaystyle{ \cos^3 x \over 3 } + C $ .

Click HERE to return to the list of problems.




SOLUTION 8 : Integrate 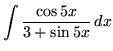$ \displaystyle{ \int {\cos {5x} \over 3 + \sin{5x} } \,dx } $ . Use u-substitution. Let

$ u = 3 + \sin {5x} $

so that (Don't forget to use the chain rule when differentiating $ \sin {5x} $.)

$ du = 5 \cos {5x} \ dx $ ,

or

$ (1/5) du = \cos 5x \ dx $ .

Substitute into the original problem, replacing all forms of $ x $, getting

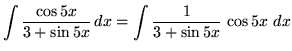
$ \displaystyle{ \int { \cos {5x} \over 3 + \sin{5x} } \,dx } = \displaystyle{ \int { 1 \over 3 + \sin{5x} } \, \cos {5x} \ dx } $

$ = \displaystyle{ \int { 1 \over u} \,(1/5)du } $

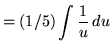
$ = \displaystyle{ (1/5) \int { 1 \over u} \, du } $

$ = (1/5) \displaystyle{ \ln \vert u\vert + C } $

$ = (1/5) \displaystyle{ { \ln { \vert 3 + \sin {5x}\vert}} + C } $ .

Click HERE to return to the list of problems.




SOLUTION 9 : Integrate 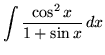$ \displaystyle{ \int { \cos^2 x \over 1 + \sin x } \,dx } $ . First use trig identity A from the beginning of this section to rewrite the function, getting

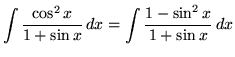
$ \displaystyle{ \int { \cos^2 x \over 1 + \sin x } \,dx } = \displaystyle{ \int { 1 - \sin^2 x \over 1 + \sin x } \,dx } $

(Now factor the numerator. Recall that $ A^2 - B^2 = (A-B)(A+B) $ .)

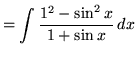
$ = \displaystyle{ \int { 1^2 - \sin^2 x \over 1 + \sin x } \,dx } $

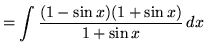
$ = \displaystyle{ \int { (1 - \sin x) (1 + \sin x) \over 1 + \sin x } \,dx}$

(Divide out the factors of $ \ 1 + \sin x $ .)

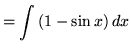
$ = \displaystyle{ \int { (1 - \sin x) } \,dx } $

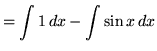
$ = \displaystyle{ \int 1 \,dx - \int \sin x \,dx } $

(Use antiderivative rule 2 from the beginning of this section.)

$ = \displaystyle{ x - (- \cos x ) + C } $

$ = \displaystyle{ x + \cos x + C } $ .

Click HERE to return to the list of problems.




Duane Kouba 2000-04-19