SOLUTION 6: $ \ \ $ Integrate $ \displaystyle{ \int {
\sqrt{1-x^2} \over x } \ dx } $. Use the trig substitution
$$ x = \sin \theta $$
so that
$$ dx = \cos \theta \ d \theta $$
Substitute into the original problem, replacing all
forms of $ x $, getting
$$ \displaystyle { \int \frac{\sqrt{1-x^{2}}}{x} \ dx = \int
\frac{\sqrt {1-\sin^{2} \theta}}{\sin \theta} \ \cos \theta \ d
\theta } $$
$$ = \displaystyle { \int \frac{\sqrt{\cos^{2} \theta}}{\sin
\theta} \ \cos \ d \theta } $$
$$ = \displaystyle { \int \frac{\cos \theta}{\sin \theta} \cos
\theta \ d \theta } $$
$$ = \displaystyle { \int \frac{\cos^{2} \theta}{\sin \theta} \ d
\theta } $$
$$ = \displaystyle { \int \frac{1-\sin^{2}\theta}{\sin \theta} \ d
\theta } $$
$$ = \displaystyle { \int (\frac{1}{\sin \theta} -\sin \theta) \ d
\theta } $$
$$ = \displaystyle { \int \Big(\csc \theta - \sin \theta \Big) \ d \theta } $$
(Recall that $ \displaystyle { \int \csc \theta \ d
\theta = \ln \Big|\csc \theta - \cot \theta \Big| + C } $)
$$ = \displaystyle { \ln \Big|\csc \theta - \cot \theta \Big| + \cos
\theta + C } $$
$\Big($ We need to write our final answer in terms of $x$.
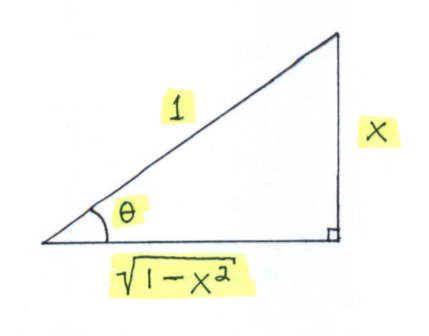
Since $ x = \sin \theta $ it follows that
$$ \sin \theta = \displaystyle{ x \over 1 } = \displaystyle{ opposite \over hypotenuse } $$
and from the Pythagorean Theorem that
$$ \displaystyle (adjacent)^2 + (opposite)^2 = (hypotenuse)^2
\ \ \longrightarrow $$
$$ (adjacent)^2 + (x)^2 = (1)^2
\ \ \longrightarrow \ \ \ adjacent = \sqrt{1-x^2} \ \ \longrightarrow $$
$$ \cos \theta = \displaystyle{ adjacent \over hypotenuse }= \displaystyle{ \sqrt{1-x^2} \over 1 } $$
$$ \cot \theta = \displaystyle{ adjacent \over opposite }= \displaystyle{ \sqrt{1-x^2} \over x } $$
and
$$ \csc \theta = \displaystyle{ hypotenuse \over opposite }= \displaystyle{ 1 \over x } . \Big) $$
$$ = \displaystyle { \ln \Big|\frac{1}{x} - \frac{\sqrt{1-x^{2}}}{x} \Big|
+ \sqrt{1-x^{2}} + C } $$
Click HERE to return to the list of problems.

